Noel Bonneuil
, Nicolas
Brouard,
Jean-Marie Robine
5th meeting of the International Network on Health Expectancy
(REVES-5): Future Uses of Health Expectancy Indices
Ottawa, February 19-20-21 1992
The paper is not finished, but most of the mathematics are here. Results will be presented by graphs
Let us describe the process with a simple example. At age 70, during the first round of the LSOA survey (1984), 348 individuals were interviewed: 333 declared to be capable of performing each of the seven activities of daily living without help, 15 did not pass and were classified as ``dependent''. During the second round, in 1986, 21 from the 333, were found in the ``disability'' status and 26 others had died. On the other hand, 6 of the initially 15 ``dependent'' individuals in 1984 recovered a healthy status, and 2 of them died (table 1).
Table: DETAILS OF THE LSOA RESULTS FOR PEOPLE AGE 70 IN 1984: STATUS IN 1986.
In 1984,the initial 333 individuals, aged 70.5 years,
could be considered as exposed to either of two competing risks: moving to the
disability status, with force 
or dying with force
. On the other hand, the force of ``recovery'' is
and the force of mortality from disbalility status is
as described by figure 1.
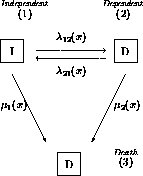
Figure 1: 4 forces are involved in the model.
Forces are supposed to vary continuously according to age.
We have a description of the situation in 1986 but we do not know the history of each individual between 1984 and 1986: we know that 26 of 333 independent individuals in 1984 died but we do not know if, in the interval, some of them have been momentarily disabled before dying from the disability status.
Before going on to the ``demographic analysis'' and the discussion on the accuracy of the estimators and of the survey, we need to describe the mathematical and statistical aspects of the process of moving between health and disability status.