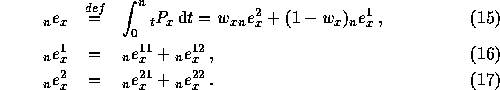The mean time spent in each state between time [0,n] is an important synthetic index. It depends of the initial age x and initial status j.
Let 
, be a random variable describing the status of
an individual at time t, if initially at age x and in status j
at time 0. We have:
If we now introduce
, is the proportion of time n
spent in status k. This again is a random variable, for which expectancy,
or ``life expectancy'' can be written:
Generally life expectancy is not enough to synthesize the whole distribution, particularly when variance is high. The variance can be computed as:
Since
we find
For symetrical reasons, one can integrate on a subdiagonal
, and this leads with 
, to:
Since the process is markovian
The variance can thus finally be written:
Other important relations, such as the disaggregation into life expectancy with disability and disability-free life expectancy can be deduced:
