...Methods

Prepared by Eric Hauet at Institut National d'Etudes Démographiques, France. This practical guide can be read in hypertext on the EURO-REVES Web server at the following URL: http:/euroreves.ined.fr/euroreves/cookdef/cookdef.html

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...table

A classical form of the life table is the complete life table. Complete life tables are based on deaths registered each year. For each age group (single age year) the number of deaths is determined and is then divided by the corresponding population size at the middle of the year to obtain an age-specific death rate. Proportion of those alive at age x dying in the interval [x,x+1[ is derived. These proportions (probabilities) are the basic quantities from which all li (i>0) and Li are computed. The estimate of the life expectancy at every age x, ex, is also provided by such a table.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...table

An abridged life table is like a complete life table but deals with age intervals greater than one year, except the first years of life. A typical set of intervals is [0,1[, [1,5[, [5,10[, [10,15[, etc.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...states

The distinguishing characteristic of a Markov process is that it is `memoryless', meaning that the state an individual occupies next depends on the state currently occupied, but not on those occupied in the past

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...large

Those who become ill get well again quickly.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...comparisons

In the particular case of dementia, see for example [11]

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...by[7]

Validity of these formulae is conditional to several hypotheses approximately verified when using life table data and when prevalence ratios are not smoothed.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...interval

Generally, we have no information about the distribution of deaths inside the interval. So, we take

67#20

which is assuming that deaths are uniformly distributed in interval .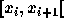

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...smaller

This approximation would be irrelevant if the the sample size of the survey is very large. In that case, let 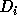the number of death registered during a year, one can use the formula (see [4] p163):

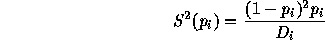
. But in that case, it is necessary to notice that the approximation of section 3.2.2 (a) deserves also to be removed.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...survey

If the sample is stratified or has required a important reweighting on individuals responding because of loss of follow up from the initial sampling until the health interview, and resampling, then it can be appropriate to use a more complex formula (which is often provided from the people doing the sampling).

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

...negligible

The following general formulae (for example, see [10] chapter 13) must be used (X and Y are assumed as random variables) and applied to formula 8:

80#26

where 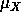is E(X) and 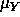is E(Y). Moreover, if X and Y are two ratios measured on the same age-group i,

.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

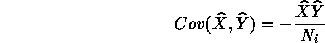
...expectancies

It is the same for the difference between Life expectancy and Health expectancy.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.